Section: New Results
Estimation in biomechanics
Exponential convergence of an observer based on partial field measurements for the wave equation
Participants : Dominique Chapelle, Philippe Moireau.
We analyze an observer strategy based on partial—that is, in a subdomain—measurements of the solution of a wave equation, in order to compensate for uncertain initial conditions. We prove the exponential convergence of this observer under a nonstandard observability condition, whereas using measurements of the time derivative of the solution would lead to a standard observability condition arising in stabilization and exact controllability. Nevertheless, we directly relate our specific observability condition to the classical geometric control condition. Finally, we provide some numerical illustrations of the effectiveness of the approach. This work in collaboration with M. de Buhan (Univ. Paris V) and N. Cîndea (Univ. Clermont-Ferrand) is published in [13] .
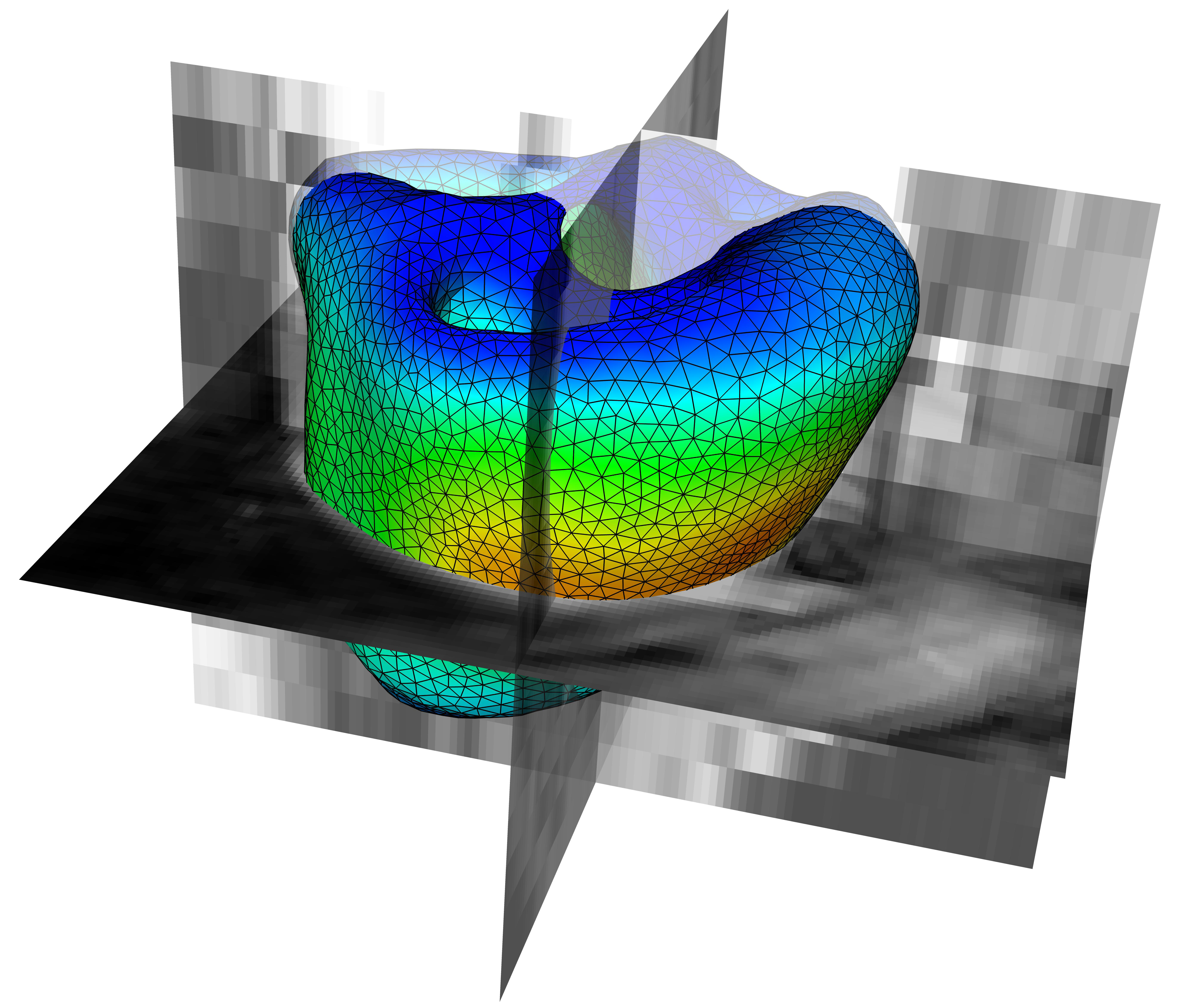
Sequential identification of boundary support parameters in a fluid-structure vascular model using patient image data
Participants : Dominique Chapelle, Philippe Moireau.
This work [17] is in collaboration with C. Bertoglio and J.‐F. Gerbeau (REO team) and N. Xiao, C.A. Figueroa and C.A. Taylor (Stanford University), where we propose a complete methodological chain for the identification of the corresponding boundary support parameters, using patient image data. We consider distance maps of model to image contours as the discrepancy driving the data assimilation approach, which then relies on a combination of (1) state estimation based on the so-called SDF filtering method, designed within the realm of Luenberger observers and well-adapted to handling measurements provided by image sequences, and (2) parameter estimation based on a reduced-order UKF filtering method which has no need for tangent operator computations and features natural parallelism to a high degree. Implementation issues are discussed, and we show that the resulting computational effectiveness of the complete estimation chain is comparable to that of a direct simulation. Furthermore, we demonstrate the use of this framework in a realistic application case involving hemodynamics in the thoracic aorta. The estimation of the boundary support parameters proves successful, in particular in that direct modeling simulations based on the estimated parameters are more accurate than with a previous manual expert calibration. This paves the way for complete patient-specific fluid-structure vascular modeling in which all types of available measurements could be used to estimate additional uncertain parameters of biophysical and clinical relevance.
This work published in BMMB (impact factor 3.192) can be considered as the first trial of data assimilation using real data in hemodynamics.
Filtering strategies using image data
Participants : Alexandre Imperiale, Philippe Moireau, Alexandre Routier.
Some progress has been achieved concerning the Luenberger filtering procedure – also known as nudging – for the cardiovascular system in several directions. We have studied the impact of data interpolation (in time and space) on the method performance (a paper on this subject is being prepared) and, during Alexandre Routier end-of-curriculum internship from INSA Rouen, we have adapted the formalism of currents (inspired by a collaboration with S. Durrleman). This formalism in an elegant way to represent geometric objects (endo- and epicardium surfaces for example) as operators on a test vector space defined on the ambient space. From this key idea the main work was to define a numerical tractable norm on the space of surfaces and derive it with respect to the Lagrangian displacement of the solid domain in order to incorporate such a representation of surfaces into our filtering technique. Among other advantages this new observer requires significantly less prior efforts in terms of image processing.
Formulation of observers for parabolic equations
Participants : Karine Mauffrey, Philippe Moireau.
We are currently working on optimal filtering using observers for a class of evolution PDEs including heat-like equations. As for the optimal control issue,the optimal filtering issue is related to the resolution of a differential Riccati equation. In [25] or [29] , the link between the optimal filtering formulations and the derived Riccati equation is done by finite dimension arguments. There exist also other results on the linear quadratic optimal control that are based on infinite dimensional considerations (see, for example, [26] and [28] ). A work in progress consists in presenting a direct approach for the optimal filtering issue, using infinite dimension considerations only. Then we should be able to introduce reduced-rank considerations to be able to stabilize only the low frequencies part of the parabolic system, and therefore offer a discretization strategy. This discretization will the be analyzed in details.